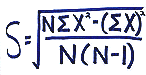
| X | X2 |
| 49 | 2401 |
| 48 | 2304 |
| 43 | 1849 |
| 39 | 1521 |
| 34 | 1156 |
| 33 | 1089 |
| 28 | 784 |
| 27 | 729 |
| 25 | 625 |
| 25 | 625 |
| 22 | 484 |
| 22 | 484 |
| 22 | 484 |
| 20 | 400 |
| 15 | 225 |
| 452 | 15160 |
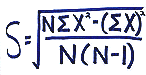
| S = | 15 (15160) - 4522 |
| 15 (15-1) |
S = 10.48718
The variance is 109.98095. The standard deviation is 10.48718. The variance is the square of the standard deviation. 10.48718 squared is equal to 109.98095.
S2 = 109.98095
The range is 34. The high score is 49; the low score is 15. 49 - 15 = 34.
Range = 34
It depends on how you look at the question. If you look at the mean of approximately 30 minutes. Then having a standard deviation of approximately 10.5 minutes seems rather large. But if you put it in the broader context, a standard deviation of approximately 10.5 minutes of study time is not all the big.